搜索结果: 1-15 共查到“偏微分方程”相关记录1918条 . 查询时间(2.828 秒)
上海科技大学数学科学研究所岳海天教授与合作者最近的两项研究成果分别发表于《数学年鉴》(Annals of Mathematics) 和《数学新进展》(Inventiones Mathematicae)，相继彻底解决了色散方程领域悬而未决近三十年的两个难题：二维高阶非线性薛定谔方程和三维三阶非线性波方程下的吉布斯测度不变性问题。
中国科学院软件所分布式SMT求解器研究工作获CAV杰出论文奖（图）
系统 结构 非线性算术
2024/9/20
2024年7月25日，中国科学院软件研究所基础软件与系统重点实验室（计算机科学国家重点实验室）的论文Distributed SMT Arithmetic Theories Solving Based on Dynamic Variable－level Partitioning在形式化验证领域国际旗舰会议Computer Aided Verification（CAV 2024）上荣获杰出论文奖（CA...
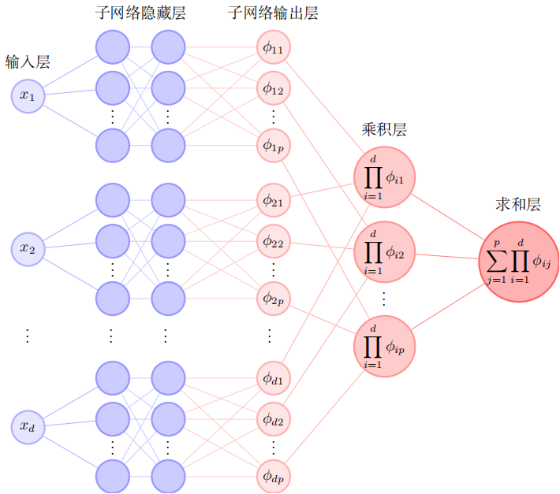
由于“维数灾难”的原因，求解高维偏微分方程一直是数学、物理、化学等学科中具有本质困难的问题。基于深度神经网络的机器学习方法为解决这一问题提供了潜在的可能性，目前已经设计了多种机器学习方法来求解高维偏微分方程。这些方法由于需要进行采样或使用Monte-Carlo方法进行高维积分来计算损失函数，往往导致求解精度远低于经典算法求解低维偏微分方程的精度，实际应用范围也受到了很大的限制。
黄淮学院数学与统计学院吴忠林教授（图）
黄淮学院数学与统计学院 吴忠林 教授 偏微分方程
2024/3/28
吴忠林，男，1972年3月生，教授、博士。1996年7月河南大学数学专业本科毕业。2006年7月河南大学基础数学专业硕士毕业。
上海外国语大学贤达经济人文学院数据科学学院硕士生导师周渊教授（图）
上海外国语大学贤达经济人文学院数据科学学院 硕士生导师 教授 随机控制 数学金融 非线性方程分析 孤立子理论 数据科
2024/3/7
周渊，教授，硕士导师。复旦大学数学系，获理学学士和理学硕士学位，留学美国南佛罗里达大学获数学与应用数学专业博士学位。
一类二维双曲混沌偏微分系统的可观性与观测器
二维双曲混沌 偏微分系统 可观性 观测器
2023/12/13
This paper is concerned with both observability and observers for a class of systems described by the two-dimensional hyperbolic PDEs with superlinear boundary conditions which can exhibit chaos. The ...
随机退化抛物方程的两类多目标问题
随机退化 抛物方程 两类多目标问题
2023/12/13
This paper is devoted to studying two multiobjective problems for stochastic degenerate parabolic equations. The first one is a hierarchical control problem, in which the controls are classified into ...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars："Inverse problems for elliptic PDE" (7)
椭圆偏微分方程 反问题 Calderón问题
2023/11/29
This course will give an introduction to inverse problems for elliptic partial differential equations. The most famous example of such problems is the Calderón problem, which arises in seismic and med...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Laplacian estimates for the cscK equation
cscK方程 拉普拉斯估计 Nash-Moser迭代
2023/11/29
We will report Chen-Cheng’s work on cscK equations. We derive the Laplacian bound using Nash-Moser iteration.
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：Analytic smoothing effect of a class of ultra-parabolic equations
一类超抛物型方程 解析 光滑效应
2023/11/29
We study a class of ultra-parabolic equations, it is a high order degenerate parabolic operators of Hormander type, so it is strongly degenerate, but we prove that this class operators possesses the a...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars："Inverse problems for elliptic PDE" (8)
椭圆偏微分方程 反问题 Calderón问题
2023/11/29
This course will give an introduction to inverse problems for elliptic partial differential equations. The most famous example of such problems is the Calderón problem, which arises in seismic and med...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars："Sobolev extensions"(7)&(8)
Sobolev扩展 Whitney扩展 技术变体
2023/11/29
I will give an introduction to Sobolev extensions, including the use of variants of the Whitney extension technique. I will concentrate on the basics of the theory.
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars："Sobolev extensions"(5)&(6)
Sobolev扩展 Whitney扩展 技术变体
2023/11/29
I will give an introduction to Sobolev extensions, including the use of variants of the Whitney extension technique. I will concentrate on the basics of the theory.
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：C1,1/3- very weak solutions to the two dimensional Monge–Ampère equation
二维 Monge–Ampère方程 C1,1/3极弱解
2023/11/29
For any θ<1/3 , we show that very weak solutions to the two-dimensional Monge–Ampère equation with regularity C1,θ are dense in the space of continuous functions. This result is shown by a convex inte...
Academy of Mathematics and Systems Science, CAS Colloquia & Seminars：An inverse Lax-Wendroff procedure for finite difference schemes on the Cartesian grids
笛卡尔网格 有限差分格式 Lax-Wendroff逆过程
2023/11/13
In this talk, we introduce the so-called inverse Lax-Wendroff (ILW) boundary treatment for finite difference approximations on the Cartesian mesh. As the domain boundary may intersect with the grid in...